Kuhn tucker conditions maximization problem

We now have two constraints. Kuhn-Tucker conditions then the point x 0is a maximum.

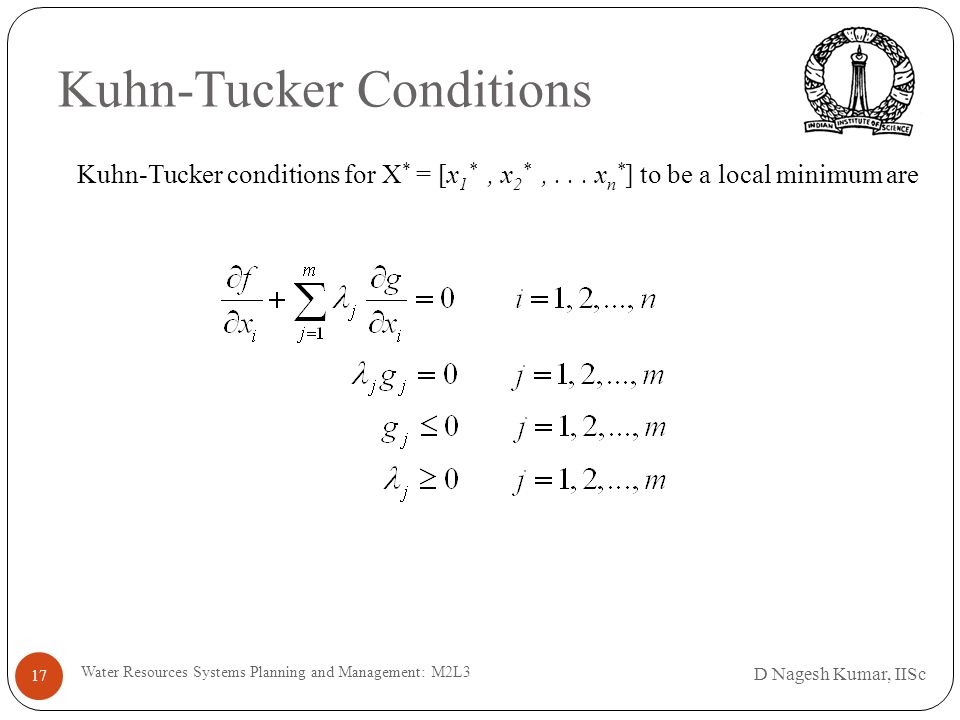
The method of Lagrange Multipliers is used to find the solution for optimization problems constrained to one or more equalities. When our constraints also have inequalities, we need to extend the method to the KKT conditions. Two numerical examples are provided for illustration. Kuhn Tucker Method - Karush Kuhn Tucker conditions (KKT) - Quadratic Programming Problem - Part - Duration: 24:30.

Kuhn–Tucker conditions to a qualitative economic analysis. There is no reason to insist that a consumer spend all her wealth. NDCQ is checked on the boundary of the constraint set. As for the equation of the isoquant, this is a separate problem, how to draw it.

Actually, if we solve for x_in terms of x_ we get. In both maximization and minimization, the Lagrange multipliers corresponding to equality constraints are unrestricted in sign.

Sufficiency of the KKT Conditions. They incorporate the restrictions implied by these constraints directly into the first-order conditions.

The KKT condition for this problem has the form For the above, we can eliminate to obtain Some possible points in that satisfy these conditions are depicted in Figure 21. For example in the general problem of maximising a strictly concave function sub-ject to x ≤ c, the conditions imply that at a maximum theslopecannotbenegative. For instance, for this particular problem.

Talk:Karush– Kuhn – Tucker conditions Jump to. Kuhn - Tucker theorem There are lots of propositions linking.

For example, minimization problems imply a different set up of conditions than maximization problems. In mathematical optimization, the Karush– Kuhn – Tucker (KKT) conditions, also known as the Kuhn – Tucker conditions, are First-order necessary conditions for a solution in nonlinear programming to be optimal, provided that some regularity conditions are satisfied.

Allowing inequality constraints, the KKT approach to nonlinear programming generalizes the method of Lagrange multipliers, which. RC (income) the consumer’sutility maximization problem is as follows: maximize u. The third condition says that either λ∗or c−g(x ∗,y) must be zero.

The problem is well-defined because B. If λ= then the constraint can be slack the FOC turn into the (Unconstrained FOC) considered in 1. If λ∗then the constraint must be binding then the problem turns into the standard Lagrangean. Stack Exchange network consists of 1QA communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.

The criteria for meeting the necessary and sufficient condition is established and verified based on Kuhn H. Caveats and Extensions Notice that we have been referring to the set of conditions which a solution to the maximization problem must satisfy. These conditions are often labeled as "–rst order conditions " or "FOCs" for the corresponding maximization problem. Isett in Calculus, economics, Uncategorized.

How many possible cases are there in this problem ? In this paper it is also introduced the use of these mathematical methods of optimization in economics. The theorem gives a set of sucient (but not necessary) conditions for a point satisfying the rst set of conditions to be optimal. Now, since γ∗ ≥and g(x∗)≤ we have ⟨γ,g(x∗)⟩≥for all γ≥with equality for γ∗ (complementary slackness).

We also have ⟨λ,h(x∗)⟩=0. Let x ∗ be a local minimum point of (). Then there exists λ = (λ. . ,λm)⊤ such that.

Optimality Conditions for Constrained Optimization Problems Robert M. Introduction Recall that a constrained optimization problem is a problem of the form (P) min x f(x) s. The latter seem redundant because the Inada conditions will guarantee an interior solution.